Playoff Percentages
Jun 21, 2017 17:48:14 GMT -8
Rob Manfred (Trade Review), Former Twins GM (Robin), and 3 more like this
Post by Rockies GM (Dan) on Jun 21, 2017 17:48:14 GMT -8
Methodology
Thanks to Griffin's system, we were able to translate my power rankings into projected W%. The numbers used were slightly different from my last Power Rankings because in this exercise, I'm not worried about what has happened, but rather what is projected to happen.
Using those W% numbers, I was able to predict the likelihood of each team winning against any other team (if you can make out the numbers here, first find the team in the column, go across to their opponent, and there you have the winning% for the first team vs the second. This article explains how the numbers are derived.
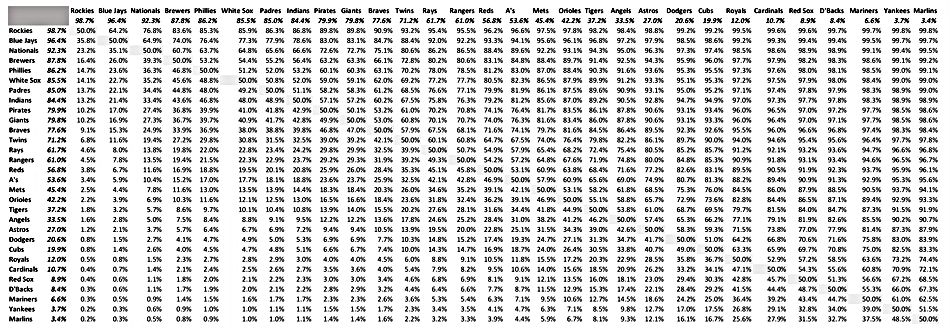
Based on those numbers, I use a Monte Carlo algorithm in order to simulate the season 100,000 times. The games that have already been played are locked in, so each season now starts from Week 12. I sim the actual schedule, so, despite the fact that one team might have a higher W%, it doesn't mean they'll win more games. Take San Diego and San Francisco for example (I just noticed them because they're in my division) - only one win separates them, but despite the fact that San Diego is actually projected for a better W%, the program has the SF widening the gap. That is because SD's ROS strength of schedule is drastically harder, with an average 58.9702 W% of their remaining opponents as opposed to SF's 42.5197%.
Results
I also made the program sim out through the playoffs and record the times each team won the Pennant and the World Series. I won't post those numbers here, since they're relatively meaningless, but some interesting tidbits:
Thanks to Griffin's system, we were able to translate my power rankings into projected W%. The numbers used were slightly different from my last Power Rankings because in this exercise, I'm not worried about what has happened, but rather what is projected to happen.
Using those W% numbers, I was able to predict the likelihood of each team winning against any other team (if you can make out the numbers here, first find the team in the column, go across to their opponent, and there you have the winning% for the first team vs the second. This article explains how the numbers are derived.

Based on those numbers, I use a Monte Carlo algorithm in order to simulate the season 100,000 times. The games that have already been played are locked in, so each season now starts from Week 12. I sim the actual schedule, so, despite the fact that one team might have a higher W%, it doesn't mean they'll win more games. Take San Diego and San Francisco for example (I just noticed them because they're in my division) - only one win separates them, but despite the fact that San Diego is actually projected for a better W%, the program has the SF widening the gap. That is because SD's ROS strength of schedule is drastically harder, with an average 58.9702 W% of their remaining opponents as opposed to SF's 42.5197%.
Results
| NL West | Division% | Wild Card % | Total Playoff % | Average Wins |
| Arizona | 0% | 0% | 0% | 6.08 |
| Colorado | 88.85% | 11.12% | 99.97% | 18.2 |
| Los Angeles | 0% | 0% | 0% | 2.48 |
| San Diego | 0.85% | 69.47% | 70.32% | 13.88 |
| San Francisco | 10.3% | 88.95% | 99.25% | 16.38 |
| NL Central | Division% | Wild Card % | Total Playoff % | Average Wins |
| Chicago | 0% | 0% | 0% | 7.44 |
| Cincinnati | 0.08% | 1.44% | 1.52% | 10.31 |
| Milwaukee | 57.7% | 38.8% | 96.5% | 15.52 |
| Pittsburgh | 42.22% | 51.27% | 93.49% | 15.24 |
| St. Louis | 0% | 0% | 0% | 4.78 |
| NL East | Division% | Wild Card % | Total Playoff % | Average Wins |
| Atlanta | 0.52% | 0.88% | 1.4% | 9.6 |
| Miami | 0% | 0% | 0% | 1.46 |
| New York | 0.41% | 0.34% | 0.75% | 9.87 |
| Philadelphia | 45.87% | 20.87% | 66.74% | 13.45 |
| Washington | 53.21% | 16.86% | 70.07% | 13.53 |
| AL West | Division% | Wild Card % | Total Playoff % | Average Wins |
| Houston | 4.09% | 0.76% | 4.85% | 9.43 |
| Los Angeles | 9.24% | 1.9% | 11.14% | 9.91 |
| Oakland | 60.47% | 4.33% | 64.8% | 12.49 |
| Seattle | 0.13% | 0.01% | 0.14% | 7.11 |
| Texas | 26.07% | 6.05% | 32.12% | 11.11 |
| AL Central | Division% | Wild Card % | Total Playoff % | Average Wins |
| Chicago | 10.39% | 53.44% | 63.83% | 12.98 |
| Cleveland | 71.23% | 25.56% | 96.79% | 15.24 |
| Detroit | 0.8% | 15.33% | 16.13% | 11.29 |
| Kansas City | 0% | 0% | 0% | 3.74 |
| Minnesota | 17.57% | 66.12% | 83.69% | 13.75 |
| AL East | Division% | Wild Card % | Total Playoff % | Average Wins |
| Baltimore | 0.03% | 47.19% | 47.22% | 12.04 |
| Boston | 0% | 0% | 0% | 2.16 |
| New York | 0% | 0% | 0% | 3.48 |
| Tampa Bay | 0.12% | 79.16% | 79.28% | 13.52 |
| Toronto | 99.85% | 0.15% | 100% | 18.54 |
I also made the program sim out through the playoffs and record the times each team won the Pennant and the World Series. I won't post those numbers here, since they're relatively meaningless, but some interesting tidbits:
- Parity - While some teams have better odds of winning the World Series than others, 19 teams won at least 5 championships (with rounding enough to equal 0.01%). Similarly, 21 teams won at least 5 league pennants
- Favorites - With that said, only three teams recorded double digit odds. The Rockies won the championship 29.93% of all simulations, the Blue Jays won 27.74% and the Indians squeaked in with 10.82%
- Most Common Matchups - The five most common World Series matchups were as follows:
- Colorado vs Toronto (23.75%)
- Colorado vs Cleveland (11.92%)
- Milwaukee vs Toronto (8.8%)
- Washington vs Toronto (4.41%)
- San Francisco vs Toronto (4.36%)
- Least Common Matchups - Out of 100,000 simulations 10 matchups only ever happened once. Those are:
- Atlanta vs Oakland
- Cincinnati vs Chicago White Sox
- Cincinnati vs Minnesota
- Cincinnati vs Oakland
- Cincinnati vs Tampa Bay
- New York Mets vs Cleveland
- New York Mets vs Minnesota
- New York Mets vs Tampa Bay
- New York Mets vs Texas
- Washington vs Houston
- Unique Matchups - Out of the 19 teams who made the World Series, there were 94 unique matchups

